
The neighbouring contraption consists of three elements:
Boggle your mind, trying to envisage building this object.
Our brain reconstructs an internal 3-dimensional world model from the flat retinal image. Locally (at each corner) the 3d-reconstruction is always possible, but globally incompatible viewpoints are combined, leading to an “impossible” object.
The oldest –known to me– example of an impossible scene: “Madonna and Child ~ Adoration of the Magi” from the Pericope of Henry II around 1025. The impossible architecture in that painting, however, seems not done purposefully to me, in contrast to Hogarth’s ‘Frontispiece’. Often the first impossible object is attributed to Reutersvard (a design for a Swedish stamp), however Albers and Hogarth were clearly earlier. After the Penroses formally described the phenomenon, examples abound, beautifully drawn for instance by Escher.
Albers, Josef (1931) “Rolled Wrongly”
Escher MC (1960) “Belvedere” or “Ascending and descending” (Andrew Lipson’s LEGO version for the latter)
Hogarth, William (1754) Satire on False Perspective
Magritte (1965) “Carte blanche”
Penrose & Penrose (1958) Impossible objects: A special type of illusion. Brit J Psychol 14(1):31–33
Reutersvard, Oscar (1934) “Opus 1” etc.
Schuster DH (1964) A new ambiguous figure: A threestick clevis. American J Psychology 77(4):673 [download at jstore]
Wu, Fu, Yeung, Yia & Tang (2010) Modeling and rendering of impossible figures. ACM Transactions on Graphics 29 13:2–13:15
More on perspective by Chris Tyler
On the left you see an animation where you can draw along if you grab a paper and a pen.
Start with 6 vertical equidistant lines, give them hats. You get a rendition of “3 towers” or a “three-pronged fork”. Obviously, a sensible 3-dimensional interpretation.
Cover the top, and draw connecting circle segments at the bottom. You see the bottom of a square bar, bent into an U-shape. Again, a sensible 3-dimensional interpretation.
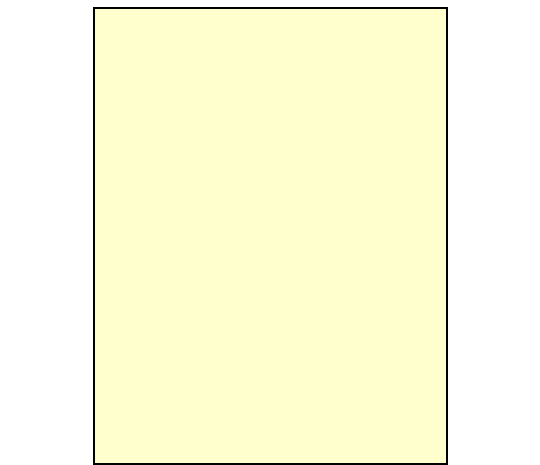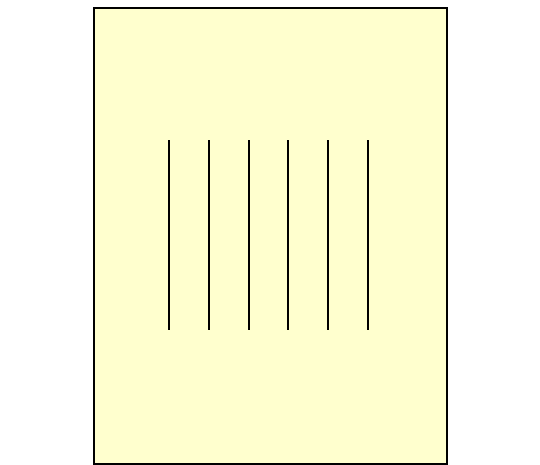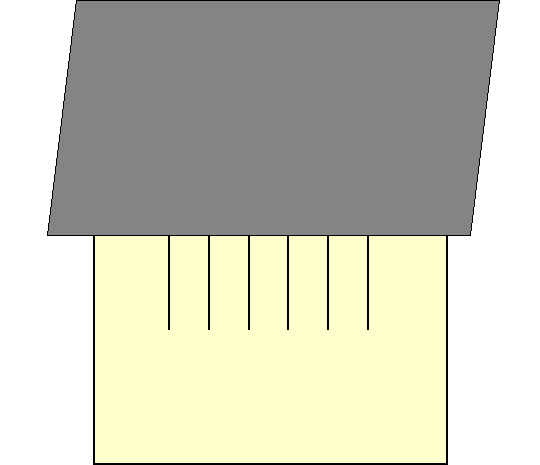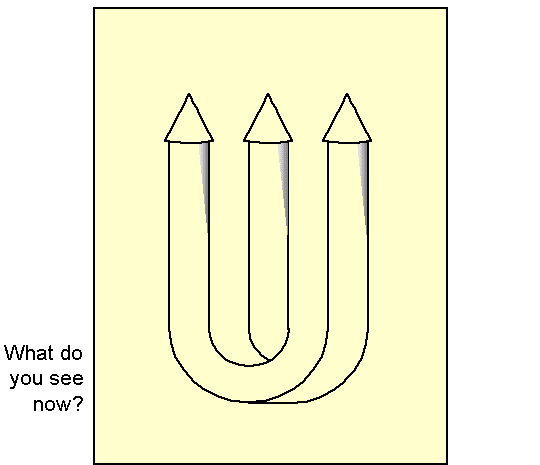
However, uncovering the top also reveals the fact that the two interpretations are not compatible with each other.